W.Y.C. Chen and Z.-G. Liu,
Parameter augmentation for basic hypergeometric series I,
In: B.E. Sagan and R.P. Stanley (eds.),
Mathematical Essays in Honor of Gian-Carlo Rota, 111-129, Birkhäuser, Boston, 1998.

Cited by

  1. M.A. Abdlhusein, The Euler operator for basic hypergeometric series, Int. J. Adv. Appl. Math. Mech. 2 (2014) 42-52.

  2. M.A. Abdlhusein, The generalized Hahn polynomials, TWMS J. Appl. Eng. Math. 5 (2015) 231-248.

  3. M.A. Abdlhusein, Two operator representations for the trivariate q-polynomials and Hahn polynomials, Ramanujan J. 40 (2016) 491-509.

  4. M.A. Abdlhusein, The new application of the Cauchy operator, Journal of Zankoi Sulaimani, Part-A (2015) 193-204.

  5. S.A. Ali and A. Agnihotri, A new summation formula for 2ψ2 basic bilateral hypergeometric series by q-exponential operator technique, Advanced Studies in Contemporary Mathematics 29(3) (2019) 331-338.

  6. S.A. Ali and A. Agnihotri, Certain basic hypergeometric series identities through q-exponential operator technique, International Bulletin of Mathematical Research 1 (2014) 49-53.

  7. S.A. Ali and A. Agnihotri, Parameter augmentation for basic hypergeometric series by Cauchy operator, Palest. J. Math. 6 (2017) 159-164.

  8. J. Cao, New proofs of generating functions for Rogers–Szegö polynomials, Appl. Math. Comput. 207 (2009) 486-492.

  9. J. Cao, Bivariate generating functions for Rogers–Szegö polynomials, Appl. Math. Comput. 217 (2010) 2209-2216.

  10. J. Cao, Notes on Carlitz's q-operators, Taiwanese J. Math. 14 (2010) 2229-2244.

  11. J. Cao, Alternative proofs of generating functions for Hahn polynomials and some applications, Infin. Dimens. Anal. Quantum Probab. Relat. Top. 14 (2011) 571-590.

  12. J. Cao, Moments for generating functions of Al-Salam-Carlitz polynomials, Abstr. Appl. Anal. (2012) Art. ID 548168, 18 pp.

  13. J. Cao, q-Difference equations for generalized homogeneous q-operators and certain generating functions, J. Difference Equ. Appl. 20 (2014) 837-851.

  14. J. Cao, A note on generalized q-difference equations for q-beta and Andrews–Askey integral, J. Math. Anal. Appl. 412 (2014) 841-851.

  15. J. Cao, Notes on Askey-Roy integral and certain generating functions for q-polynomials, J. Math. Anal. Appl. 409 (2014) 435-445.

  16. J. Cao, Homogeneous q-partial difference equations and some applications, Adv. in Appl. Math. 84 (2017) 47-72.

  17. J. Cao and Z.-H. Shen, On q-integral representations for q-Hahn and Askey-Wilson polynomials and certain generating functions, Appl. Math. E-Notes 17 (2017) 186-198.

  18. J. Gao, B.B. Xu, S. Arjika, A note on generalized q-difference equations for general al-salam–carlitz polynomials, arXiv:2011.05848v1.

  19. J. Cao and X.-L. Zhao, Exponential operator decomposition for Carlitz type generating functions, Ars Combin. 116 (2014) 245-255.

  20. W.Y.C. Chen and A.M. Fu, Cauchy augmentation for basic hypergeometric series, Bull. Lond. Math. Soc. 36 (2004) 169-175.

  21. W.Y.C. Chen and A.M. Fu, Semi-finite forms of bilateral basic hypergeometric series, Proc. Amer. Math. Soc. 134 (2006) 1719-1725.

  22. W.Y.C. Chen and Z.-G. Liu, Parameter augmentation for basic hypergeometric series, II, J. Combin. Theory Ser. A 80 (1997) 175-195.

  23. V.Y.B. Chen and N.S.S. Gu, The Cauchy operator for basic hypergeometric series, Adv. in Appl. Math. 41 (2008) 177-196.

  24. A. Di Bucchianico and D. Loeb , A selected survey of umbral calculus, Electron. J. Combin. 2 (2000) Dynamic Survey 3, 34 pp.

  25. T. Ernst, The history of q-calculus and a new method, Department of Mathematics, Uppsala University, 2000.

  26. T. Ernst, q-Calculus as operational algebra, Proc. Est. Acad. Sci. 58 (2009) 73-97.

  27. T. Ernst, Examples of a q-umbral calculus, Adv. Stud. Contemp. Math. (Kyungshang) 16 (2008) 1-22.

  28. T. Ernst, Handbuch für die q-Analysis, Springer International Publishing Switzerland, 2012.

  29. J.-P. Fang, q-Differential operator identities and applications, J. Math. Anal. Appl. 332 (2007) 1393-1407.

  30. J.-P. Fang, A note on the Rogers-Fine identity, Electron. J. Combin. 14 (2007) Note 17, 5 pp.

  31. J.-P. Fang, Extensions of q-Chu-Vandermonde's identity, J. Math. Anal. Appl. 339 (2008) 845-852.

  32. J.-P. Fang, Remarks on a generalized q-difference equation, J. Difference Equ. Appl. 21 (2015) 934-953.

  33. N.S.S. Gu, On the bilateral series , 中国科技论文在线, 2012.

  34. V.J.W. Guo, Elementary proofs of some q-identities of Jackson and Andrews–Jain, Discrete Math. 295 (2005) 63-74.

  35. Z.Y. Jia, Two new q-exponential operator identities and their applications, J. Math. Anal. Appl. 419 (2014) 329-338.

  36. Z.Y. Jia, A new extension of the nonterminating 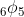summation via q-difference equation, Taiwanese J. Math., to appear.

  37. C. Krattenthaler and K.S. Rao, Automatic generation of hypergeometric identities by the beta integral method, J. Comput. Appl. Math. 160 (2003) 159-173.

  38. A. Kumar, M.S. Khan and K.P. Yadav, Parameter augmentation for some basic hypergeometric series identities, J. Math. Comput. Sci. 2 (2012) 1532-1538.

  39. N.N. Li and W. Tan, Two generalized q-exponential operators and their applications, Adv. Difference Equ. (2016) Paper No. 53, 14 pp.

  40. Z.-G. Liu, Some operator identities and q-series transformation formulas, Discrete Math. 265 (2003) 119-139.

  41. Z.-G. Liu, Two q-difference equations and q-operator identities, J. Difference Equ. Appl. 16 (2010) 1293-1307.

  42. Z.-G. Liu, An extension of the non-terminating summation and the Askey–Wilson polynomials, J. Difference Equ. Appl. 17 (2011) 1401-1411.

  43. Z.-G. Liu, On the q-partial differential equations and q-series, In: The Legacy of Srinivasa Ramanujan, 213-250, Ramanujan Math. Soc. Lect. Notes Ser. 20, Ramanujan Math. Soc. Mysore, 2013.

  44. Z.-G. Liu and J. Zeng, Two expansion formulas involving the Rogers–Szegö polynomials with applications, Int. J. Number Theory 11 (2015) 507-525.

  45. D.-Q. Lu, q-difference equation and the Cauchy operator identities, J. Math. Anal. Appl. 359 (2009) 265-274.

  46. Y.-P. Mu, Parameter augmentation and the q-Gosper algorithm, J. Symbolic Comput. 43 (2008) 874-882.

  47. K.N. Murthy, A study of the theory of basic hypergeometric series and allied topics, Ph.D. Thesis, University of Mysore, 2013.

  48. D.-W. Niu and L. Li, q-Laguerre polynomials and related q-partial differential equations, J. Difference Equ. Appl. 24 (2018) 375-390.

  49. H.L. Saad and M.A. Abdlhusein, The q-exponential operator and generalized Rogers-Szegö polynomials, J. Adv. Math. 8 (2014) 1440-1455.

  50. H.L. Saad and A.A. Sukhi, The q-exponential operator, Appl. Math. Sci. 7 (2005) 6369-6380.

  51. D.D. Somashekara, K.N. Murthy, and S.L. Shalini, On a new summation formula for basic bilateral hypergeometric series and its applications, Int. J. Math. Math. Sci. 2011 2011) Art. ID 132081, 7 pp.

  52. H.M. Srivastava and M.A. Abdlhusein, New forms of the Cauchy operator and some of their applications, Russ. J. Math. Phys. 23 (2016) 124-134.

  53. M.J. Wang, Generalizations of Milne's U(n+1) q-binomial theorems, Comput. Math. Appl. 58 (2009) 80-87.

  54. Y.-P. Wang and J.Z. Yang, Two q-series identities from Heine transformation formula summation, 洛阳师范学院学报 30 (2011) 5-7.

  55. C.H. Zhang, Parameter augmentation for two formulas, Electron. J. Combin. 13 (2006) Note 19, 5 pp.

  56. C.H. Zhang and Z.Z. Zhang, Extensions of two q-series identities, Adv. Stud. Contemp. Math. (Kyungshang) 13 (2006) 81-85.

  57. Z.Z. Zhang, A note on an identity of Andrews, Electron. J. Combin. 12 (2005) Note 3, 3 pp.

  58. Z.Z. Zhang, Some 3ψ3 transformations formulas related to Bailey's 2ψ2, Ars Combin. 78 (2006) 257-265.

  59. Z.Z. Zhang, Operator identities and several U(n+1) generalizations of the Kalnins–Miller transformations, J. Math. Anal. Appl. 324 (2006) 1152-1167.

  60. Z.Z. Zhang and M.X. Liu, Applications of operator identities to the multiple q-binomial theorem and q-Gauss summation theorem, Discrete Math. 306 (2006) 1424-1437.

  61. Z.Z. Zhang and J. Wang, Two operator identities and their applications to terminating basic hypergeometric series and q-integrals, J. Math. Anal. Appl. 312 (2005) 653-665.

  62. Z.Z. Zhang and T.Z. Wang, Operator identities involving the bivariate Rogers–Szegö polynomials and their applications to the multiple q-series identities, J. Math. Anal. Appl. 343 (2008) 884-903.

  63. Z.Z. Zhang and J.Z. Yang, Several q-series identities from the Euler expansions of and , Arch. Math. (Brno) 45 (2009) 47-58.

  64. Z.Z. Zhang and J.Z. Yang, On two identities of Fu and Lascoux, Adv. Stud. Contemp. Math. (Kyungshang) 18 (2009) 59-67.

  65. Y. Zhou and Q.M. Luo, Some new generating functions for q-Hahn polynomials, J. Appl. Math. 2014 (2014) Art. ID 419365, 5 pp.

  66. J.-M. Zhu, The solutions of four q-functional equations, arXiv:1001.0299.

  67. 张之正, 杨继真, 双参数有限 q 指数算子及其应用, 数学学报 53 (2010) 1007-1018.

  68. H.W.J. Zhang, (q,c)-Derivative operator and its applications, Adv. in Appl. Math. 121 (2020) 102081.

    69.